講座主題：基于局部Kelvin-Voigt型阻尼的波方程的鎮(zhèn)定問題
專家姓名：韓忠杰
工作單位：天津大學
講座時間：2022年1月4日 14:30-15:30
講座地點：騰訊會議,，會議ID：654-328-245
主辦單位：煙臺大學數(shù)學與信息科學學院
內(nèi)容摘要：
The stabilization of a multi-dimensional wave equation on cuboidal domain is considered. By applying the localized Kelvin-Voigt damping, in a region where the Geometric Control Condition (GCC) is not satisfied, we prove that the system can be polynomially stabilized to 0 with the polynomial decay rate
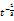
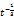under smooth initial conditions. Especially, the optimality of this explicit decay rate is verified for this system through a careful resolvent estimate. We further consider the case that the damping coefficient is degenerated at the interface. An explicit decay rate which is dependent on the degeneration of the damping coefficient is further estimated.
主講人介紹：
天津大學數(shù)學學院教授,，從事分布參數(shù)系統(tǒng)控制理論方面的研究,。主要成果發(fā)表在SIAM Journal on Control and Optimization, IEEE Transactions on Automatic Control, ESAIM -Control Optimisation and Calculus of Variations等控制理論相關(guān)期刊,，主持國家自然科學基金3項（完成2項，在研1項）,。
